
In addition to the AB topics in this unit, BC students analyze polar and vector-valued functions.
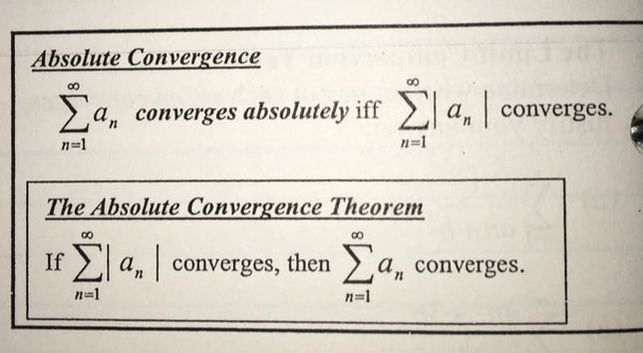
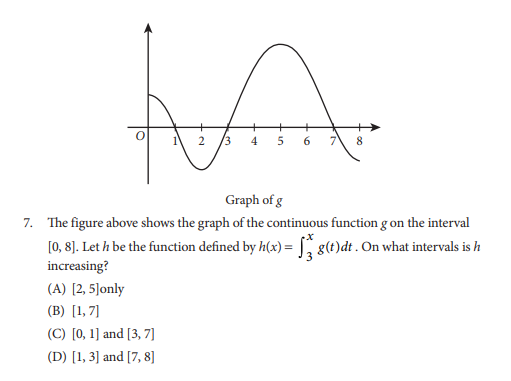
#Sequences and series ap calculus bc how to
Students learn how to use calculus to model and analyze changing aspects of our world. This unit focuses on Second Derivatives and Applications of Derivatives within Topic II: Derivatives of the College Board's Calculus BC topic outline. Sums, Differences, Products, and Quotients.Students also interpret the derivative as a rate of change and move fluidly between multiple representations including graphs, tables, and equations. They learn techniques for finding derivatives of algebraic functions (such as y = x2) and trigonometric functions (such as y = sin x). Students learn how to calculate a derivative, the slope of a curve at a specific point. This unit addresses Topic II: Derivatives of the College Board's Calculus BC topic outline. Intermediate and Extreme Value Theorems.Continuity is an important property of functions. Limits help students understand differentiation (the slope of a curve) and integration (the area inside a curved shape). Students learn two important concepts that underlie all of calculus: limits and continuity. This unit addresses Topic I: Functions, Graphs, and Limits of the College Board's Calculus BC topic outline. They learn what calculus is, why it was invented, and what it is used for. Students prepare to study calculus by reviewing some basic pre-calculus concepts from algebra and trigonometry. Students prepare for the AP Exam and further studies in science, engineering, and mathematics.Ĭourse Outline SEMESTER ONE Unit 1: The Basics

Students also learn to understand change geometrically and visually (by studying graphs of curves), analytically (by studying and working with mathematical formulas), numerically (by seeing patterns in sets of numbers), and verbally. Students learn to evaluate the soundness of proposed solutions and apply mathematical reasoning to real-world models. Calculus helps scientists, engineers, and financial analysts understand the complex relationships behind real-world phenomena. In this course, students study functions, limits, derivatives, integrals, and infinite series. This course is the equivalent of an introductory college-level calculus course.


 0 kommentar(er)
0 kommentar(er)
